Kuhrt AB-2 and A2
I thought I might illustrate one of the lesser known and used mechanisms for mechanical calculation at the hand of one of the four machine types that used it. The machines are, to the best of my knowledge, the experimental Brunsviga T, which was never series-produced, the Kuhrt, the Alpina and the Numeria machines. Instead of the regular Odhner pinwheel, in which the pins move outwards due to the action of a setting disk, they have so called "axial pinwheels", or in German "Schwenksprossenräder", in which the pins that actuate the numeral wheels move out from the disk sideways instead of radially. One of the machines in my collection is a Kuhrt type "AB 2", which has the top plate with slits for the backtransfer mechanism, but the mechanism itself has either been removed or never been assembled into this machine. The holes for mounting it are all tapped, so maybe a subsequent removal because something broke makes the most sense. The serial number is 1924. Machine N° 1925 is illustrated on rechnerlexicon, it is a Kuhrt AB-5, with the backtransfer mechanism, and a 10 row keyboard.N° 1924 carries a builder plate on the back that says "Deutsche Rechenmaschinen-Werke A.G. Leipzig, TYPE AB 2", and a distributor plate on the top reading: "L'application du calcul mécanique, 42 Av. Berthélot, LYON, Tél PARM. 03.41". The place is now a hairdresser.
The other machine was bought in the Brussels area, and is in far more original condition. Its serial number is 1561, it is a model A 2, and it has a number of missing features that are there in the AB machine. First some general overview pictures, the machine with the text on the green keyboard base is the Kuhrt A:




Both machines are very light in operation, and N° 1924 has a number of very useful features, such as coupled clearing of the result and revolution counter (which is also very light), a very nice tabbed mechanism for carriage movement (1561 has this too, obviously), very easy manual setting of values in the register, and of course, originally, a backtransfer mechanism. What it doesn't have is tens' carry in the counter register - the numbers turn red on subtraction. Both machines have a sum function, where the keyboard resets after every turn of the crank. This mechanism is engaged by pushing the keyboard clearing lever over to the right. In its leftmost position, the keyboard remains as it is for multiplication and division. The crank itself of N° 1924 has a quick release mechanism, by twisting the tabs on the side of the crank, it simply comes off and can be pocketed. This would however not prevent anyone from using the machine, because it runs so light, that the resulting stub can still easily be turned with two fingers.
Removable crank:



Coupled clearing:


Some other differences between the machines are the location of the comma pointer rail, and the presence of two filled and painted input windows in the later machine


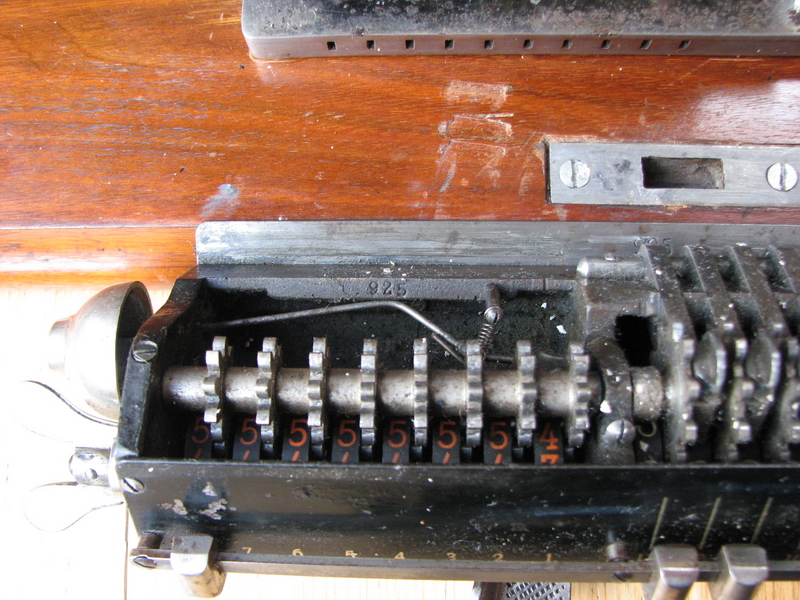
And then down to the nitty-gritty. How does an axial pinwheel work ? It can best be compared with the tens' carry in an Odhner calcualtor with regular (radial) pinwheels.


To make the process really clear, I have added a video below. You can see how a metal part is pulled to the front of the machine, the higher the key pressed, the further it is pulled. This part has a wedge on it, that engages with the teeth sticking out to the side on the pins in the axial pinwheel. Each subsequent tooth is a little lower on its respective pin. This means that the pin with the lowest tooth (1) will be pushed into the wheel when rotating past the wedge when it is barely pulled to the front. If the wedge is further out, the two lowest pins will be pushed back into the wheel, etc etc until when the 9 key is pressed, all pins are pushed into the wheel. It is the pins that move sideways into the wheel that engage with the result mechanism. There are no springs, centrifugal force or gravity to move them after they have been pushed in, so they are reset by another wedge set into the bottom of the machine, which pushes all of the pins back out, ready for a new calculation. For subtractions - the lever connected to the keys has another wedge at the back, which operates in much the same manner as the one in the front. Notice however how rotating in the other direction the order of the pins is reversed - the number is right, but adding e.g. 6 and sutracting 6 is done by different pins in the pinwheel. Tens' carry is only present in the result register, and operates in exactly the same way as in a regular pinwheel machine, and thus also in the same way as the calculating pins here - the extra tens' carry pins are pushed to the inside of the wheel to engage with the result mechnism by a little lever that reaches down from the carriage when a numeral wheel goes past 9.
Enjoy the video:
